
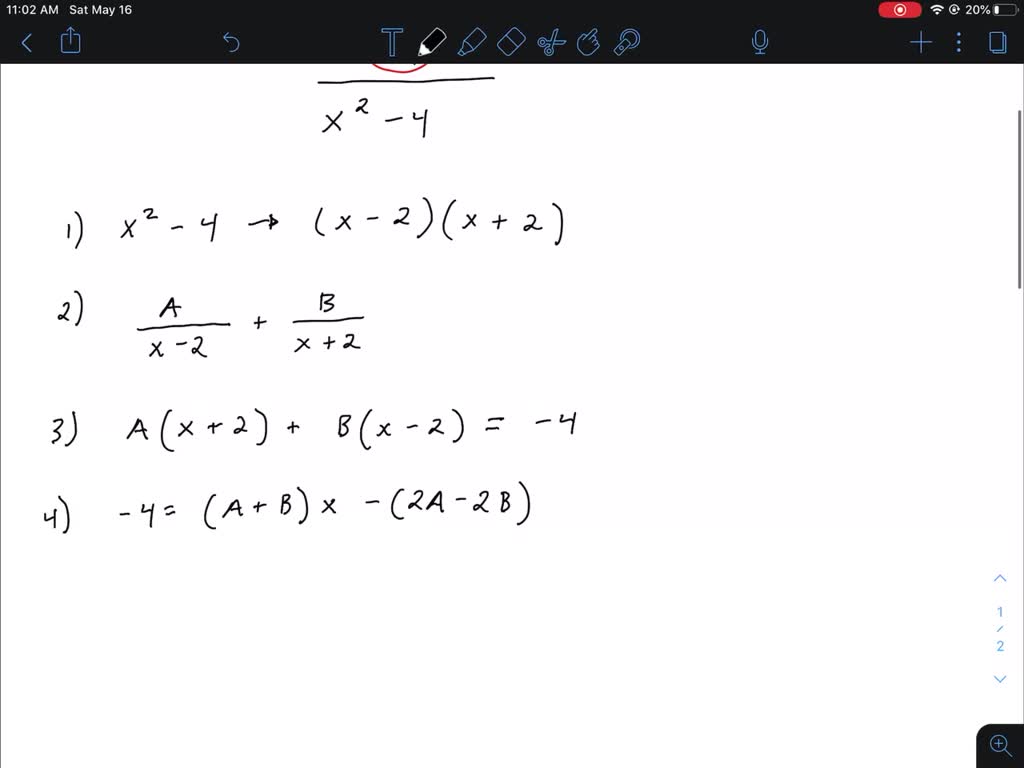
Here are the basic Partial Fraction Rules when for decomposing fractions. Basic Partial Fraction Decomposition Rules If an integral is improper (the degree of the numerator is greater than or equal to the degree of the denominator), use polynomial long division to get a term that’s not a rational function, and then decompose the remaining terms we’ll show an example below. We’ll see that when we do this, we’ll usually end up with integrating logarithmic functions or integrating inverse trigonometric functions. The basic idea is to factor the denominator (if it isn’t already factored) of the complicated factor, and then break it up into different fractions with denominators of those factors. In more advanced cases, you may need to use Completing the Square or another method to get the rational in the right form). (Note that Partial Fraction Decomposition may really only be used with rationals with denominators that factor well. Note that Integration by Partial Fractions is used when U-Substitution Integration doesn’t work easily a rule of thumb is to try U-sub first!
#Decompose into partial fractions calculator how to#
You may have learned how to use this technique in your Algebra class, and it’s quite useful in Calculus! Basically, we are breaking up one “complicated” fraction into several different “less complicated” fractions. Integration by Partial Fraction Decomposition is a procedure where we can “decompose” a proper rational function into simpler rational functions that are more easily integrated. Integration by Partial Fractions with Higher Degrees.Example of Rational Function where Partial Fractions are not Needed.Integration by Partial Fractions with Improper Fractions.Basic Partial Fraction Decomposition Rules.Introduction to Integration by Partial Fractions.Applications of Integration: Area and Volume.Exponential and Logarithmic Integration.Riemann Sums and Area by Limit Definition.Differential Equations and Slope Fields.Antiderivatives and Indefinite Integration, including Trig Integration.Derivatives and Integrals of Inverse Trig Functions.

Exponential and Logarithmic Differentiation.Differentials, Linear Approximation, and Error Propagation.Curve Sketching, including Rolle’s Theorem and Mean Value Theorem.Implicit Differentiation and Related Rates.Equation of the Tangent Line, Tangent Line Approximation, and Rates of Change.Basic Differentiation Rules: Constant, Power, Product, Quotient, and Trig Rules.Differential Calculus Quick Study Guide.Polar Coordinates, Equations, and Graphs.Law of Sines and Cosines, and Areas of Triangles.Linear and Angular Speeds, Area of Sectors, and Length of Arcs.Conics: Circles, Parabolas, Ellipses, and Hyperbolas.Graphing and Finding Roots of Polynomial Functions.Graphing Rational Functions, including Asymptotes.Rational Functions, Equations, and Inequalities.Solving Systems using Reduced Row Echelon Form.The Matrix and Solving Systems with Matrices.Advanced Functions: Compositions, Even and Odd, and Extrema.Solving Radical Equations and Inequalities.Solving Absolute Value Equations and Inequalities.Imaginary (Non-Real) and Complex Numbers.Solving Quadratics by Factoring and Completing the Square.Introduction to Multiplying Polynomials.Scatter Plots, Correlation, and Regression.Algebraic Functions, including Domain and Range.Systems of Linear Equations and Word Problems.Introduction to the Graphing Display Calculator (GDC).Direct, Inverse, Joint and Combined Variation.Coordinate System and Graphing Lines, including Inequalities.Types of Numbers and Algebraic Properties.


 0 kommentar(er)
0 kommentar(er)
